Aim: To determine the viscosity of benzene using Ostwald’s U-tube viscometer.
Principle: The viscosity of a fluid is the internal resistance to flow. This is also called the coefficient of dynamic viscosity.
The relationship between the flow rate of a liquid through a capillary tube and the viscosity is the principle behind the determination of Newtonian fluids (fluids exhibiting streamlined flow). This relationship is expressed in Poiseuille’s equation:
V=\frac{P\pi r^4t}{8l.\eta}\;or\;\eta=\frac{P\pi r^4t}{8l.V}
Where
V = Volume of liquid (cc) flowing in time t (second).
P = Driving pressure (dyne /cm2).
r = Radius of the capillary (cm).
l = Length of the capillary (cm).
\eta= Coefficient of viscosity (poise).
When the P is due to the force of gravity, then P = h p g, where h is the height of the liquid, p is the density of the liquid and g is the acceleration due to gravity.
Now, \eta=\frac{h\rho g\pi r^4t}{8l.V}
When the equal volume of two liquids (one test and the other reference standard whose viscosity is known) is flown through the same apparatus under the same driving pressure,
Then \frac{\;\eta_{test}}{\;\eta_{s\tan dard}}=\frac{\;\rho_{test}\;.\;t_{test}\;}{\rho_{s\tan dard\;}.\;\rho_{s\tan dard}} as other terms: h,g, r, l, and V are equal for both test and standard and get canceled. As absolute viscosity determination is difficult, the viscosity of a liquid can be determined easily by comparing it with a liquid whose viscosity is known using the above equation written as \eta_{test}=\eta_{s\tan dard}\times\frac{\;\rho_{test}\;.\;t_{test}\;}{\rho_{s\tan dard\;}.\;\rho_{s\tan dard}} and water can be used as a reference liquid whose viscosity is known.
The Ostwald’s U-tube viscometer is used for the determination of the viscosity of Newtonian fluids.
Materials and apparatus required: Ostwald’s U tube viscometer, pipette, stopwatch, density measurement facility, benzene, and water.
Procedure:
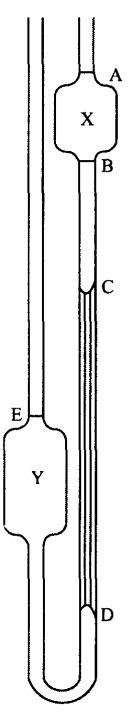
- The cleaned viscometer is vertically clamped.
- Sufficient liquid whose viscosity is to be determined is poured using a pipette into bulb Y to reach mark E.
- The liquid is then sucked or blown up to a point viscometer 1 cm above A.
- The time for the liquid to fall from A to B is measured using a stopwatch. This is repeated three times to ensure that accurate time is only recorded.
- The density of the liquid is measured using a specific gravity bottle.
- In a similar way, the data for the standard liquid (water) is recorded using the same apparatus after cleaning and drying.
Observation and Calculation:
Room Temperature: ____ 0C.
Time for the liquid to fall from A to B (t test) = ________seconds.
The density of the liquid (determined separately) (r test) = __________g/cc.
Time for water to fall from A to B (t standard) = ___________seconds.
The density of water (determined separately/can be obtained from appendix at room temperature) (r standard) = ______g/cc. The viscosity of the liquid =\eta_{test}=\eta_{s\tan dard}\times\frac{\;\rho_{test}\;.\;t_{test}\;}{\rho_{s\tan dard\;}.\;\rho_{s\tan dard}}
The viscosity of water at room temperature can be obtained from the standard table (refer to appendix).
The unit of viscosity as calculated in poise can be converted to SI units by multiplying the value by 10-1.
Report: The viscosity of the liquid (benzene) at room temperature (specify) is Pa.s. (the usual value is 5.6 mPa.s)
Viscosities of some liquids at 20°C
| Liquid | Viscosity in mPa.s (centipoises) |
| Benzene | 5.6 at 30 oC |
| Chloroform | 0.58 |
| Castor oil | 986 |
| Ethanol | 1.20 |
| Glycerol | 1490 |
| Water | 1.002 |
Make sure you also check our other amazing Article on: Determination of Specific Surface Area
